Gamma is one of those terms that seems to pop up in multiple disciplines—physics, mathematics, finance, and even computer science. But one question that often comes up is: Can gamma be zero? This seemingly simple question leads to fascinating discussions across different fields.
Gamma is a term used across multiple scientific and financial fields, and while its meaning changes depending on the context, it always represents some kind of rate of change, transformation, or function. Before we get into whether gamma can be zero, let’s first understand what it is in different disciplines.
Gamma in Physics
In physics, gamma (𝛾) commonly appears in relativity and radiation physics:
- In Special Relativity:
- The Lorentz factor (𝛾) is given by: γ=11−v2/c2\gamma = \frac{1}{\sqrt{1 – v^2/c^2}}
- It describes how time, length, and mass change at speeds close to the speed of light.
- Can gamma be zero here? Not really, because that would imply an impossible scenario where velocity v is greater than the speed of light (which breaks the laws of physics).
- In Electromagnetic Radiation:
- Gamma rays are high-energy electromagnetic waves with frequencies above 101910^{19} Hz.
- These are emitted in nuclear reactions, radioactive decay, and even cosmic explosions.
- Can gamma rays have zero energy? Theoretically, no. If gamma rays had zero energy, they wouldn’t be gamma rays at all!
Gamma in Mathematics
In mathematics, the most famous appearance of gamma is in the Gamma function (Γ), which extends the concept of factorials to real and complex numbers:
Γ(n)=∫0∞xn−1e−xdx\Gamma(n) = \int_0^\infty x^{n-1} e^{-x} dx
- Can gamma be zero in math? Yes, but only under certain conditions. The Gamma function (Γ(n)) is zero at negative integers and zero.
Gamma in Finance
In financial markets, particularly options trading, gamma measures how much an option’s delta (rate of price change) shifts as the underlying asset moves.
- If gamma = 0, it means the option’s delta is not changing at all, which can happen in deep in-the-money or deep out-of-the-money options.
| Field | Definition of Gamma | Can Gamma Be Zero? |
|---|---|---|
| Physics (Relativity) | Time dilation factor | No, it would require exceeding light speed |
| Physics (Radiation) | High-energy EM waves | No, zero-energy gamma rays wouldn’t exist |
| Mathematics | Gamma function (Γ) | Yes, at certain values |
| Finance | Sensitivity of delta in options | Yes, in rare cases |
So, the answer to “Can gamma be zero?” depends on the field. In physics, it’s generally no, while in mathematics and finance, it’s possible under certain conditions.
Gamma in Physics: Can Gamma Be Zero?
Physics is one of the most fascinating fields where gamma plays a crucial role. Whether we’re talking about Einstein’s theory of relativity or high-energy gamma rays, gamma appears in equations that shape our understanding of the universe. But can gamma ever be zero in physics? Let’s explore!
Gamma in Special Relativity: The Lorentz Factor
One of the most famous uses of gamma in physics is in Einstein’s theory of special relativity. The Lorentz factor, represented by 𝛾 (gamma), describes how time, length, and mass behave at speeds close to the speed of light:
γ=11−v2/c2\gamma = \frac{1}{\sqrt{1 – v^2/c^2}}
Where:
- v = velocity of an object
- c = speed of light (≈ 299,792,458 m/s)
Can Gamma Be Zero in Special Relativity?
Let’s do some quick math:
- If v = 0 (an object at rest), then 𝛾 = 1, meaning no relativistic effects.
- If v = c (an object moving at the speed of light), then we get division by zero, which is mathematically undefined.
- For gamma to be zero, we’d need 1−v2/c2=∞1 – v^2/c^2 = \infty, which is impossible!
Gamma can never be zero in special relativity. The only values it can take are 𝛾 ≥ 1, with no possibility of dropping to zero.
What If Gamma Were Zero?
If 𝛾 = 0, it would imply that time dilation and length contraction are infinite, and objects could travel faster than light. This directly contradicts Einstein’s laws of physics, which state that nothing with mass can exceed the speed of light.
Gamma Rays: Can They Have Zero Energy?
Now let’s switch gears and talk about gamma rays—those extremely powerful waves zipping through the universe. Gamma rays have frequencies higher than 101910^{19} Hz and carry massive amounts of energy.
Can Gamma Rays Have Zero Energy?
Energy of a photon (including gamma rays) is given by:
E=hfE = h f
Where:
- E = energy of the photon
- h = Planck’s constant (6.626×10−346.626 × 10^{-34} Js)
- f = frequency of the wave
For gamma rays to have zero energy, their frequency would have to be zero. But at zero frequency, the wave does not exist!
Gamma rays can never have zero energy because that would mean they don’t exist at all.
| Concept | Definition of Gamma | Can Gamma Be Zero? |
|---|---|---|
| Lorentz Factor (Relativity) | Time dilation factor | ❌ No, would require faster-than-light travel |
| Gamma Rays (Radiation) | High-energy EM waves | ❌ No, zero energy means no gamma rays exist |
Final Thoughts on Gamma in Physics
- In relativity, gamma is always ≥ 1 and never zero.
- In gamma radiation, a zero-energy gamma ray would simply not exist.
- If gamma could be zero, we’d be living in a universe where time doesn’t exist as we know it—something straight out of science fiction!
Gamma in Mathematics: Can Gamma Be Zero?
Mathematics is where gamma takes on a completely different identity. Unlike in physics, where gamma is often a factor tied to motion or energy, in math, gamma is a function—one with deep connections to calculus, number theory, and even probability. But can gamma be zero in mathematics? Let’s break it down!
The Gamma Function (Γ): A Supercharged Factorial
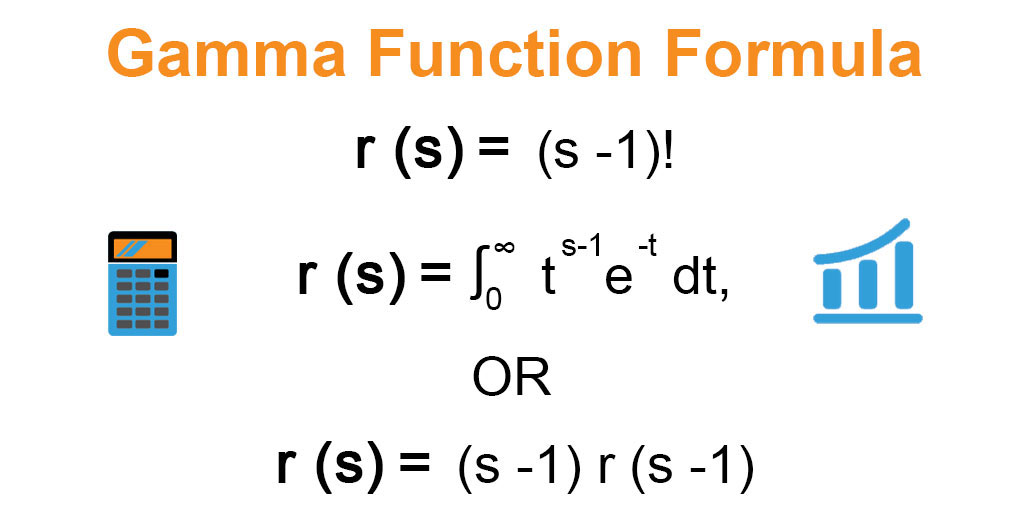
One of the most famous appearances of gamma in mathematics is in the Gamma function (Γ), which generalizes the factorial to real and complex numbers. It is defined as:
Γ(n)=∫0∞xn−1e−xdx\Gamma(n) = \int_0^\infty x^{n-1} e^{-x} dx
For positive integers, the Gamma function satisfies:
Γ(n)=(n−1)!\Gamma(n) = (n-1)!
For example:
- Γ(5)=4!=4×3×2×1=24\Gamma(5) = 4! = 4 × 3 × 2 × 1 = 24
- Γ(3)=2!=2×1=2\Gamma(3) = 2! = 2 × 1 = 2
This function is widely used in statistics, physics, and engineering, particularly in probability distributions like the Gamma and Beta distributions.
Can the Gamma Function Be Zero?
The Gamma function never equals zero for positive numbers, but it does have zeros at certain points in the negative domain. Specifically, Gamma(0) and all negative integers (-1, -2, -3, etc.) are undefined or infinite, meaning that Gamma has simple poles at these points.
| Value of n | Γ(n) Output |
|---|---|
| Γ(5) | 24 |
| Γ(3.5) | 3.323 |
| Γ(1) | 1 |
| Γ(0) | Undefined (Singularity) |
| Γ(-1) | Undefined (Singularity) |
| Γ(-2.5) | Finite Negative Value |
The Gamma function never equals zero for positive numbers, but it does have singularities (undefined points) at zero and negative integers. However, it does not have actual zeros in the typical sense.
The Digamma Function: Where Gamma’s Derivative Might Be Zero
A related function, the digamma function (ψ), is the derivative of the natural logarithm of the Gamma function:
ψ(x)=ddxlnΓ(x)\psi(x) = \frac{d}{dx} \ln \Gamma(x)
Unlike the Gamma function itself, the digamma function does have real zeros, which occur at specific points known as the roots of the digamma function. The first zero occurs at approximately x ≈ 1.46.
Does Gamma Ever Truly Equal Zero in Math?
| Mathematical Concept | Can Gamma Be Zero? |
|---|---|
| Gamma Function (Γ(n)) | ❌ No, but it is undefined at 0 and negative integers |
| Digamma Function (ψ(n)) | ✅ Yes, has real zeros at specific values |
Final Thoughts on Gamma in Mathematics
- The Gamma function (Γ(n)) itself never equals zero but is undefined at 0 and negative integers.
- The digamma function (ψ(n)), which is the derivative of the Gamma function, does have zeros at certain values.
- If you’re ever in an advanced math class and someone asks, “Can gamma be zero?”—you can confidently answer, “Not in the Gamma function, but in its derivative!”

Gamma in Finance: Can Gamma Be Zero?
In finance, gamma plays a critical role in options trading, where it measures how much an option’s delta changes as the underlying asset’s price moves. This might sound complicated, but don’t worry—I’ll break it down in simple terms, with some real-world examples.
Understanding Gamma in Options Trading
First, let’s define a few key concepts:
- Delta (Δ): Measures how much the price of an option changes for every $1 move in the underlying asset.
- Gamma (Γ): Measures how much delta changes as the underlying asset moves.
- Theta (Θ): Measures how much an option’s price declines as time passes (time decay).
Gamma is important because it helps traders understand the rate of acceleration of delta—in other words, how sensitive an option’s price is to changes in the underlying asset.
How Gamma Works in Practice
Let’s say you buy a call option on Apple stock (AAPL), which is currently trading at $150.
- Your option’s delta is 0.50, meaning the option gains $0.50 for every $1 increase in Apple’s stock price.
- If gamma is 0.05, this means that if Apple’s stock rises to $151, delta will increase from 0.50 to 0.55.
Gamma is highest when an option is at the money (ATM)—when the stock price is close to the strike price. As the option moves deep in the money (ITM) or out of the money (OTM), gamma decreases.
Can Gamma Be Zero in Finance?
Theoretically, yes, gamma can be zero in certain cases. Here are a few scenarios where this happens:
1. Deep In-The-Money (ITM) or Deep Out-Of-The-Money (OTM) Options
- Options that are deep in the money (very profitable) or deep out of the money (unlikely to be exercised) have very low gamma, sometimes approaching zero.
- Why? Because delta is either close to 1 (for ITM options) or 0 (for OTM options), meaning it won’t change much.
2. Far Out Expiration Dates
- Options with a very long expiration period (LEAPS, for example) can have near-zero gamma because their delta changes very slowly over time.
3. Hedged Portfolios (Gamma Neutral Strategies)
- Some professional traders create gamma-neutral strategies, where they adjust their positions so that gamma is close to zero.
- This means their portfolio is less sensitive to market volatility, making it safer but limiting large profits.
| Scenario | Can Gamma Be Zero? | Why? |
|---|---|---|
| Deep ITM or OTM Options | ✅ Yes | Delta is stable, so gamma approaches zero |
| Far Out Expiration | ✅ Yes | Long-term options have slow-changing delta |
| Gamma-Neutral Strategies | ✅ Yes | Traders adjust portfolios to minimize gamma |
Final Thoughts on Gamma in Finance
- Gamma is rarely zero but can be very close to zero in specific scenarios.
- Traders manage gamma to control risk, especially in volatile markets.
- If you hear a trader say, “Gamma is near zero,” it usually means they’re holding deep ITM/OTM options or a gamma-neutral portfolio.